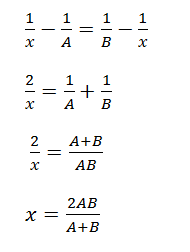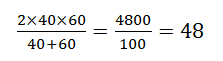One of my very favorite students greeted me this new year with a question: what are the different kinds of means? This is a fun question to explore. We are going to look at three kinds of means, each associated with a type of sequence.
Suppose we start with two numbers. Their mean is a number that lies between them. But exactly where it lies depends on what type of mean you are talking about.
If left unspecified, the term “mean” probably refers to the “arithmetic” mean. So let’s start with that one. (And by the way, helpful note for beginners: “arithmetic” here is an adjective. Read with emphasis on 3rd syllable.)
ARITHMETIC SEQUENCES AND ARITHMETIC MEANS
A sequence of numbers is called arithmetic if the difference between any two consecutive terms is a constant. For example:
2, 5, 8, 11, 14… has a common difference, d = 3. (We calculate the current term minus the previous term.)
Say we have been given two numbers A and B. We want to insert a number between them so that the three of them together form one of these arithmetic sequences. We are looking for the “arithmetic mean.”
We have A, x, B and we want the differences to be constant.
That means we can write: x – A = B – x
From that we get: 2x = A + B
And then: x = (A + B)/2
This probably does not surprise you. The arithmetic mean is also just the average.
If A = 30 and B = 60 we get an arithmetic mean of 45, thus forming the sequence:
30, 45, 60
Then, if you check, you find the difference between consecutive terms is constant:
45 – 30 = 60 – 45 = 15. So the sequence is arithmetic. 45 is the arithmetic mean between 30 and 60.
Sometimes, this is the kind of mean we care about. For example…
You drive for two hours at 30 mph and for two hours at 60 mph. What is your average speed for the trip?
When you solve this, you will discover that when you drive at different speeds for equal time periods, your average for the trip is the arithmetic mean of the two speeds.
ANOTHER SEQUENCE, ANOTHER MEAN
A geometric sequence is one where the ratio between every two consecutive terms is constant. For example:
1, 3, 9, 27, 81… is a geometric sequence with a common ratio of 3. (Now we calculate the current term divided by the previous term.)
So again we have been given two numbers A and B. And now we want to insert a number between them so that the three of them together form one of these geometric sequences. We are looking for the “geometric mean.”
We have A, x, B and we want the RATIOS to be constant.
That means we can write: x /A = B/ x
Cross multiply to get: x2 = AB
And then: x = √(AB)
For example, if A=9 and B=16 then the geometric mean x = √(9×16) =√144 = 12.
We should check our work to see if the ratios are constant:
12/9 = 16/12 = 1.333… so yes, the sequence is geometric. 12 is the geometric mean of 9 and 16.
Sometimes, this is the kind of mean we care about. For example, here is a problem you may have seen in geometry:
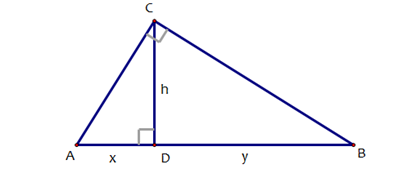
In the triangle above, if x = 9 and y = 16 determine the height, h.
You can use similar triangles to show that the height of that altitude is equal to the geometric mean of the lengths of the two segments on the hypotenuse.
ONCE MORE, WITH HARMONY
A sequence is called “harmonic” if there is a common difference between the reciprocals of consecutive terms. For example (in fact, the classic example):
1, 1/2 , 1/3, 1/4, 1/5… is harmonic. Its reciprocals are 1, 2, 3, 4, 5… which is clearly arithmetic with a common difference of 1.
And one more time, we have been given two numbers A and B. Now we want to insert a number between them so that the three of them together form one of these harmonic sequences. We are looking for the “harmonic mean.”
We have A, x, B and we want the difference of the reciprocals to be constant.
That means we can write:
So suppose A = 40 and B = 60. Their harmonic mean is:
To check our work, we have to look at the sequence of the reciprocals:
1/40, 1/48, 1/60… or, expressed over a common denominator,
6/240, 5/240, 4/240…sure enough, the reciprocals form an arithmetic sequence.
Sometimes, this is the kind of mean we care about. For example, here is an SAT classic:
You drive to work at 40 mph and then you drive back home at 60 mph. What is your average speed for the trip?
You can solve this by calling the length of each leg d, finding the time for each leg of the journey, adding to get the total time and then using total time and total distance to find average speed. When you are done, you are in for a surprise. When you travel equal distances at two different speeds, your average speed for the trip is the HARMONIC mean of the separate speeds.
I’ll close with a question: why is this called “harmonic”? Does it have something to do with music and harmony?
Happy New Year!